[암호학](-)[RSA 구현 1]

 

이론 

 

암호학 중심 이론 정리

https://verybigsilver.tistory.com/55

[암호학 둘러보기](8)[RSA]

 

수론 중심 이론 정리

https://verybigsilver.tistory.com/100

 

참고

 

 

구현

 

일단 코드를 대충 짜면 이렇게 된다.

def extended_gcd(a, b):
    if b == 0:
        return 1, 0  # 해는 (1, 0)
    x1, y1 = extended_gcd(b, a % b)  # 재귀 호출
    x = y1
    y = x1 - (a // b) * y1
    return x, y



# user parameter
# p, q는 소수
p = 73
q = 97
msg = 1615

m = p*q
phi = (p-1)*(q-1)
k = 1789
print("공개: ", m, k)

# encoding
Ciphered = msg**k%m
print(Ciphered)

# decoding
u, v = extended_gcd(k, phi)
DeCiphered = Ciphered**u%m
print(DeCiphered)

위 코드에서는 p, q, k를 직접 정해줘야 한다.
이걸 자동화 하고 암호화와 복호화를 다른 파일에서 하게 했다

#Cipher

import random
import time

# ax+by=1 해 구하는 함수
def extended_gcd(a, b):
    if b == 0:
        return 1, 0  # 해는 (1, 0)
    x1, y1 = extended_gcd(b, a % b)  # 재귀 호출
    x = y1
    y = x1 - (a // b) * y1
    return x, y

# 소수 판정 함수
def is_prime(x):
    if x < 2:
        return False
    for i in range(2, int(x**0.5) + 1):
        if x % i == 0:
            return False
    return True

# n 이상의 가장 작은 소수 찾는 함수
def next_prime(n):
    while True:
        if is_prime(n):
            return n
        n += 1

# 서로소 판정 함수
def is_coprime(a, b):
    def gcd(x, y):
        while y:
            x, y = y, x % y
        return x
    return gcd(a, b) == 1

# phi와 서로소인 k값 정하는 함수
def random_coprime(n, phi):
    while True:
        k = random.randint(2, n-1)  # 1은 너무 허접하니까 패스♡
        if is_coprime(k, phi) == 1:
            return k

# 랜덤으로 소수 p, q 만드는 함수
def random_p_q(hardness):
    rand_p_start = random.randint(1, hardness)*hardness # (0~hardness-1까지 무작위 수 뽑기)
    rand_q_start = random.randint(1, hardness)*hardness
    p = next_prime(rand_p_start)
    q = next_prime(rand_q_start)
    return p, q

random.seed(time.time())

# parameter
hardness = 10 # 암호의 난도
msg = (int)(input("보낼 메세지(숫자)를 입력하시오: "))

p, q = random_p_q(hardness)
m = p*q
phi = (p-1)*(q-1)
k = random_coprime(hardness, phi)

print("공개 파라미터\nm = %d, k = %d" %(m, k))

# encoding
Ciphered = msg**k%m
print("암호문 = ",Ciphered)

#Decipher
def extended_gcd(a, b):
    if b == 0:
        return 1, 0  # 해는 (1, 0)
    x1, y1 = extended_gcd(b, a % b)  # 재귀 호출
    x = y1
    y = x1 - (a // b) * y1
    return x, y

def prime_factor(m):
    for i in range(2, int(m ** 0.5) + 1):
        if m % i == 0:
            return i, m // i
    return None  # 소수인 경우 -> 실패

# parameter 
m = (int)(input("m값 입력: "))
k = (int)(input("k값 입력: "))
Ciphered = (int)(input("암호문 입력: "))

# decoding

# phi를 구하기 위해 소인수 분해를 해야 됨...
p, q = prime_factor(m)
phi = p*q - (p+q) + 1
u, v = extended_gcd(k, phi)
u = u%phi # <- u가 음수인 경우 처리
DeCiphered = Ciphered**u%m
print("복호문 = ", DeCiphered)

hardness에 따라 소수의 크기가 커지므로 더 어렵게 된다.

실제 프로그램을 돌려보면
hardness = 10일때

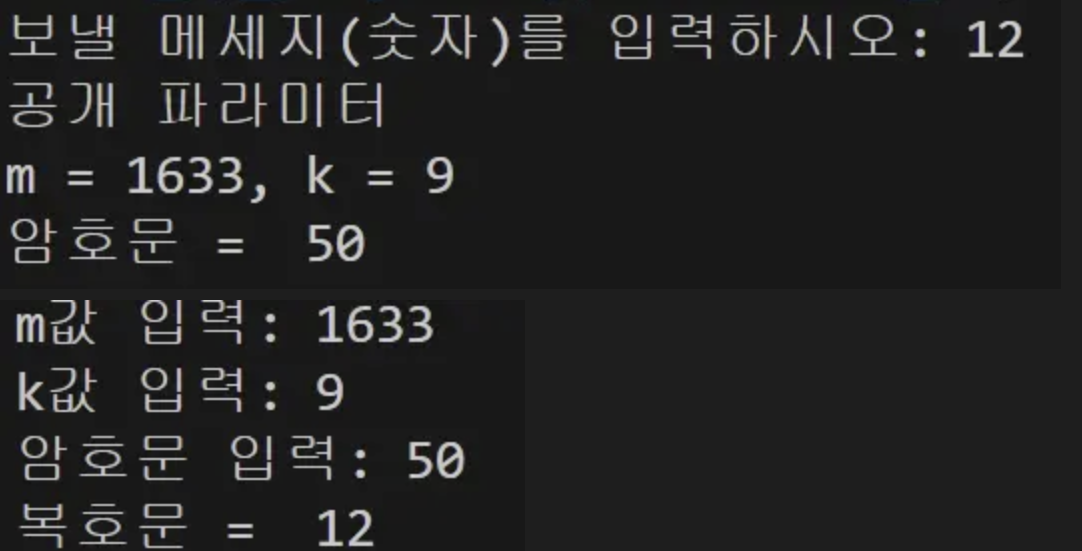
금방 복구된다.

걸리는 시간도 출력하게 코드를 수정하고
hardness 에 따라 복구되는 시간을 보면,

 

hardness	시간 (파이썬)	시간 (c)
50	0.1904008388519287 초	0.000000 초
70	0.9146127700805664 초	0.000000 초
100	인내심의 한계	0.000000 초
300	인내심의 한계	0.001000 초
500	안함	오버플로우

솔직히 파이썬이 많이 느리긴 해서 그냥 c로 옮겨서 더 돌려봤다.
그리고 큰 숫자에 대해서는 오버플로우 등의 이유로 제대로 복호화가 안될 수도 있으니 숫자는 항상 넉넉하게 잡아놓아야 한다.
근데 내가 사용하는 visual studio는 unsigned long long이 제일 큰 숫자라서 hardness가 500이상이면 오버플로우가 난다.